Numbers


Colours
August 5, 2019

National Animal of India
August 6, 2019


A number is a mathematical object used to count, measure and label. The examples are the natural numbers 1,2,3,4, and so forth. A written symbol like “5” that represents a number is called a numeral.
Here we will see numbers from 1 to 20.
| Numbers | In words |
|---|---|
  |
One |
  |
Two |
  |
Three |
  |
Four |
  |
Five |
  |
Six |
  |
Seven |
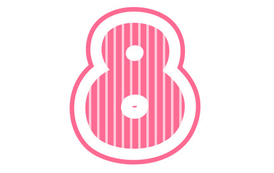  |
Eight |
  |
Nine |
  |
Ten |
  |
Eleven |
  |
Twelve |
  |
Thirteen |
  |
Fourteen |
  |
Fifteen |
  |
Sixteen |
  |
Seventeen |
  |
Eighteen |
  |
Nineteen |
  |
Twenty |
Classification of numbers:
The major categories of numbers are as follows:
| Symbol | Category | Example |
|---|---|---|
| N | Natural | 1, 2, 3, 4, 5, … |
| Z | Integer | …, −5, −4, −3, −2, −1, 0, 1, 2, 3, 4, 5, … |
| Q | Rational | Rational numbers include the integers (…, – 2, – 1, 0, 1, 2, …) and Fractions. |
| R | Real | All the rational and irrational numbers. |
| C | Complex | a + bi where a and b are real numbers and ‘i’ is a formal square root of −1. |
Colours also express emotions.
| Subclass | Definition | Example |
|---|---|---|
| Even numbers | An even number is an integer that is “divisible” by two. | 2, 4, 6, 8, 10, 12, so on. |
| Odd numbers | An odd number is an integer that is not even. | 1, 3, 5, 7, 9, so on. |
| Prime numbers | A prime number is an integer greater than 1 that is not the product of two smaller positive integers. | 2, 3, 5, 7, 11, so on. |
Calculations with numbers:
Calculation with numbers are done with arithmetical operations.
Following are the arithmetic operations:


| Arithmetic operation | Symbol | Description |
|---|---|---|
| Addition |   |
Addition is the most basic operation of arithmetic. Addition combines two quantities into a single quantity, or sum. For example, say you have a group of 2 boxes and another group of 3 boxes.If you combine both groups together, you now have one group of 5 boxes i.e. 2+3=5. |
| Subtraction |   |
Subtraction is the opposite of addition. Here we remove one quantity from another to find the difference between the two. Suppose you have a group of 5 boxes. If you then remove 3 boxes from that group, you are left with 2 boxes i.e. 5-3 = 2. |
| Multiplication |   |
Multiplication is the result of repeated additions of two numbers. The product of 5 and 3 is the result of 5 added together 3 times (5+5+5=15). Thus,multiplication of5and3 equals 15 i.e. 5×3=15. |
| Division |   |
Division is the inverse of multiplication. Here we divide a quantity into a smaller value, called the quotient. If we divide a group of 8 boxes into 4 equal groups results in 4 groups of 2 boxes i.e. 8÷4=2. |
DMAS rule:
This rule is actually order of operation which tells us which operator and which operation must be solved first in an equation.
This rule is often referred as DMAS (abbreviation of Division, Multiplication, Addition, Subtraction). It means in an equation you have to solve Division and Multiplication first, Addition and Subtraction second.
This is how its order goes
- Division.
- Multiplication.
- Addition.
- Subtraction.
Why we use DMAS rule:
Without DMAS rule all mathematical equations will come up with different answers.
Maths would become extremely hard.
It was just important to set a sequence of operation.
For Example,
Simplify: 24 – 4 ÷ 2 x 3
Solution:
24 – 4 ÷ 2 x 3
[Here order is expressed in short as ‘DMAS’ where ‘D’ stands for division, ‘M’ for multiplication, ‘A’ for addition and, ‘S’ for subtraction]
= 24 – 2 x 3 [Performing division – 4 ÷ 2 = -2]
= 24 – 6 [Performing multiplication 2 x 3 = 6]
= 18. [Performing subtraction 24 – 6 = 18]
Answer: 18.
Why we use DMAS rule:
- 2 is the only even prime number.
- 6 is the smallest perfect number, meaning it can be made by summing its divisors.
- Zero is an even number.
- 7 is the most popular “favourite number”.





